Page 44 - 4263

Analytic geometry on a plane

raising to the square power both equations and having added with each other,
2
2
2
we will get a well-known equation of a circle: x + y = τ . ,

6.4. Straight line on a plane. Its equation

The straight line is one of the main subjects of analytic geometry. Let’s consider the main types
of equations of the straight line.

6.4.1. General equation of a line

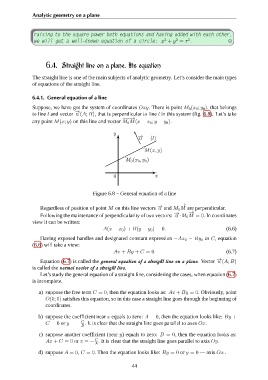
Suppose, we have got the system of coordinates Oxy. There is point M 0 (x 0 ; y 0 ), that belongs
−→
to line l and vector n (A; B), that is perpendicular to line l in this system (fig. 6.8). Let’s take
−−−→
any point M(x; y) on this line and vector M 0 M(x − x 0 ; y − y 0 ).
y
− →
n (l)

M(x, y)

M 0 (x 0 , y 0 )

. . . . . . . .
0 x

Figure 6.8 – General equation of a line

−→
−→
Regardless of position of point M on this line vectors a and M 0 M are perpendicular.
−→
−→
Following the maintenance of perpendicularity of two vectors: a ·M 0 M = 0. In coordinates
view it can be written:
A(x − x 0 ) + B(y − y 0 ) = 0. (6.6)

Having exposed handles and designated constant expression −Ax 0 − By 0 as C, equation
(6.6) will take a view:
Ax + By + C = 0. (6.7)

−→
Equation (6.7) is called the general equation of a straight line on a plane. Vector n (A; B)
is called the normal vector of a straight line.
Let’s study the general equation of a straight line, considering the cases, when equation (6.7)
is incomplete.

a) suppose the free term C = 0, then the equation looks as: Ax + By = 0. Obviously, point
O(0; 0) satisfies this equation, so in this case a straight line goes through the beginning of
coordinates.

b) suppose the coefficient near x equals to zero: A = 0, then the equation looks like: By +
C
C = 0 or y = − . It is clear that the straight line goes parallel to axes Ox.
B
c) suppose another coefficient (near y) equals to zero: B = 0, then the equation looks as:
C
Ax + C = 0 or x = − . It is clear that the straight line goes parallel to axis Oy.
A
d) suppose A = 0, C = 0. Then the equation looks like: By = 0 or y = 0 — axis Ox .

44