Page 42 - 4263

Analytic geometry on a plane

6.2.2. Turning of axes

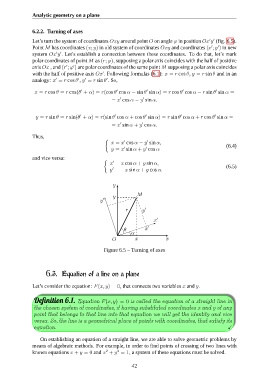
Let’s turn the system of coordinates Oxy around point O on angle φ in position Ox y (fig. 6.5).
′ ′
′
Point M has coordinates (x; y) in old system of coordinates Oxy and coordinates (x ; y ) in new
′
system Ox y . Let’s establish a connection between these coordinates. To do that, let’s mark
′ ′
polar coordinates of point M as (r; φ), supposing a polar axis coincides with the half of positive
axis Ox , and (r ; φ ) are polar coordinates of the same point M supposing a polar axis coincides
′
′
with the half of positive axis Ox . Following formulas (6.1): x = r cos θ, y = r sin θ and in an
′
analogy: x = r cos θ , y = r sin θ . So,
′
′
′
′
′
′
′
′
x = r cos θ = r cos(θ + α) = r(cos θ cos α − sin θ sin α) = r cos θ cos α − r sin θ sin α =
′
′
′
= x cos α − y sin α.
′
′
′
′
′
y = r sin θ = r sin(θ + α) = r(sin θ cos α + cos θ sin α) = r sin θ cos α + r cos θ sin α =
′
′
= x sin α + y cos α.
Thus,
{
x = x cos α − y sin α,
′
′
(6.4)
′
y = x sin α + y cos α
′
and vice versa:
{
′
x = x cos α + y sin α,
(6.5)
y = −x sin α + y cos α.
′
y
M
y
y ′
r y ′
x ′
θ x ′
. . . . . . . . . . . . . . α
O x x
Figure 6.5 – Turning of axes

6.3. Equation of a line on a plane

Let’s consider the equation: F(x, y) = 0, that connects two variables x and y.

Definition 6.1. Equation F(x, y) = 0 is called the equation of a straight line in
the chosen system of coordinates, if having substituted coordinates x and y of any
point that belongs to that line into that equation we will get the identity and vice
versa. So, the line is a geometrical place of points with coordinates, that satisfy its
equation. ✓

On establishing an equation of a straight line, we are able to solve geometric problems by
means of algebraic methods. For example, in order to find points of crossing of two lines with
2
2
known equations x + y = 0 and x + y = 1, a system of these equations must be solved.
42