Page 46 - 4263

Analytic geometry on a plane

y

(l)

−→
s
n
M 0 α
y 0
m

α . . . . . . . . . . . . .
0 x 0 x

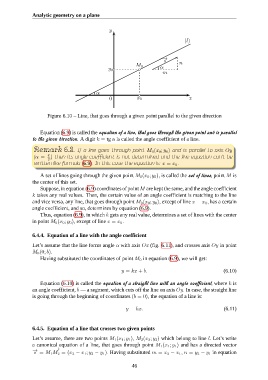
Figure 6.10 – Line, that goes through a given point parallel to the given direction

Equation (6.9) is called the equation of a line, that goes through the given point and is parallel
to the given direction. A digit k = tg α is called the angle coefficient of a line.

Remark 6.2. If a line goes through point M 0 (x 0 ; y 0 ) and is parallel to axis Oy
(α = π ), then its angle coefficient is not determined and the line equation can’t be
2
written like formula (6.9). In this case the equation is: x = x 0 .

A set of lines going through the given point M 0 (x 0 ; y 0 ), is called the set of lines, point M is
the center of this set.
Suppose, in equation (6.9) coordinates of point M are kept the same, and the angle coefficient
k takes any real values. Then, the certain value of an angle coefficient is matching to the line
and vice versa, any line, that goes through point M 0 (x 0 ; y 0 ), except of line x = x 0 , has a certain
angle coefficient, and so, determines by equation (6.9).
Thus, equation (6.9), in which k gets any real value, determines a set of lines with the center
in point M 0 (x 0 ; y 0 ), except of line x = x 0 .

6.4.4. Equation of a line with the angle coefficient
Let’s assume that the line forms angle α with axis Ox (fig. 6.11), and crosses axis Oy in point
M 0 (0; b).
Having substituted the coordinates of point M 0 in equation (6.9), we will get:

y = kx + b. (6.10)

Equation (6.10) is called the equation of a straight line with an angle coefficient, where k is
an angle coefficient, b — a segment, which cuts off the line on axis Oy. In case, the straight line
is going through the beginning of coordinates (b = 0), the equation of a line is:

y = kx. (6.11)

6.4.5. Equation of a line that crosses two given points

Let’s assume, there are two points M 1 (x 1 ; y 1 ), M 2 (x 2 ; y 2 ) which belong to line l. Let’s write
a canonical equation of a line, that goes through point M 1 (x 1 ; y 1 ) and has a directed vector
−−−−→
−→
s = M 1 M 2 = (x 2 − x 1 ; y 2 − y 1 ). Having substituted m = x 2 − x 1 , n = y 2 − y 1 in equation

46