Page 49 - 4263

Distance from the point to the line

{ 2 2
(µA) + (µB) = 1,
two properties of a normal equation are true: From the first condition:
µC < 0.
1
µ = √ (6.15)
2
± A + B 2
The sign can be chosen from the second condition: it must be opposite to the sign of a free
term in a general equation when C ̸= 0. The sign of µ is arbitrary in case C = 0. Digit µ is
called as a normal multiplier.

6.6. Distance from the point to the line

−→
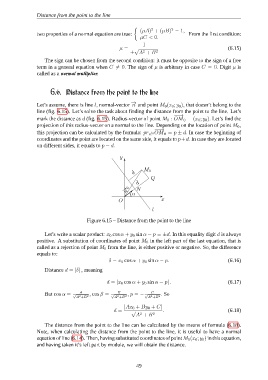
Let’s assume, there is line l, normal-vector n and point M 0 (x 0 ; y 0 ), that doesn’t belong to the
line (fig. 6.15). Let’s solve the task about finding the distance from the point to the line. Let’s
−−→
mark the distance as d (fig. 6.15). Radius-vector of point M 0 : OM 0 = (x 0 ; y 0 ). Let’s find the
projection of this radius-vector on a normal to the line. Depending on the location of point M 0 ,
−−→
this projection can be calculated by the formula: pr−→ 0OM 0 = p ± d. In case the beginning of
n
coordinates and the point are located on the same side, it equals to p+d. In case they are located
on different sides, it equals to p − d.

y

d M 0
Q
d
− 0 n N
→
. . . . . . . . . . . . α
O x

l
Figure 6.15 – Distance from the point to the line

Let’s write a scalar product: x 0 cos α + y 0 sin α − p = ±d. In this equality digit d is always
positive. A substitution of coordinates of point M 0 in the left part of the last equation, that is
called as a rejection of point M 0 from the line, is either positive or negative. So, the difference
equals to:
δ = x 0 cos α + y 0 sin α − p. (6.16)
Distance d = |δ| , meaning

d = |x 0 cos α + y 0 sin α − p| . (6.17)

A B C
But cos α = √ , cos β = √ , p = − √ . So
2
2
2
A +B 2 A +B 2 A +B 2
|Ax 0 + By 0 + C|
d = √ . (6.18)
2
A + B 2
The distance from the point to the line can be calculated by the means of formula (6.18).
Note, when calculating the distance from the point to the line, it is useful to have a normal
equation of line (6.14). Then, having substituted coordinates of point M 0 (x 0 ; y 0 ) in this equation,
and having taken it’s left part by module, we will obtain the distance.

49