Page 47 - 4263

Straight line on a plane. Its equation

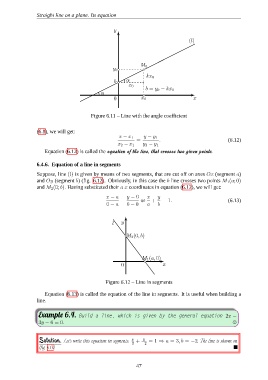
y

(l)

M 0
y 0
kx 0
b α
x 0
b = y 0 − kx 0
α . . . . . . . . . . . . . .
0 x 0 x

Figure 6.11 – Line with the angle coefficient

(6.8), we will get:
x − x 1 y − y 1
= . (6.12)
x 2 − x 1 y 2 − y 1
Equation (6.12) is called the equation of the line, that crosses two given points.

6.4.6. Equation of a line in segments

Suppose, line (l) is given by means of two segments, that are cut off on axes Ox (segment a)
and Oy (segment b) (fig. 6.12). Obviously, in this case the b line crosses two points M 1 (a; 0)
and M 2 (0; b). Having substituted their a x coordinates in equation (6.12), we will get:

x − a y − 0 x y
= or + = 1. (6.13)
0 − a b − 0 a b

l y

M 2 (0, b)

. . . . . . . M 1 (a, 0)
0 x

Figure 6.12 – Line in segments

Equation (6.13) is called the equation of the line in segments. It is useful when building a
line.

Example 6.4. Build a line, which is given by the general equation 2x −
3y − 6 = 0. ,

y
Solution. Let’s write this equation in segments: x 3 + −2 = 1 ⇒ a = 3, b = −2. The line is shown on
(fig. 6.13).

47