Page 45 - 4263

Straight line on a plane. Its equation

e) suppose B = 0, C = 0. Then the equation looks like: Ax = 0 or x = 0 — axis Oy .

Conclusion 6.1
Any equation of the first degree relatively variables x and y is the equa-
tion of the straight line on a plane and vice versa. ♢

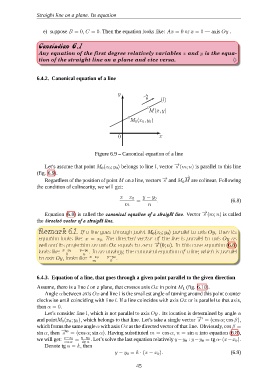
6.4.2. Canonical equation of a line

y
→ s (l)
−

M(x, y)

M 0 (x 0 , y 0 )

. . . . . . . .
0 x

Figure 6.9 – Canonical equation of a line

−→
Let’s assume that point M 0 (x 0 ; y 0 ) belongs to line l, vector s (m; n) is parallel to this line
(fig. 6.9).
−→
−→
Regardless of the position of point M on a line, vectors s and M 0 M are colinear. Following
the condition of colinearity, we will get:
x − x 0 y − y 0
= . (6.8)
m n
−→
Equation (6.8) is called the canonical equation of a straight line. Vector s (m; n) is called
the directed vector of a straight line.

Remark 6.1. If a line goes through point M 0 (x 0 ; y 0 ) parallel to axis Oy, then its
equation looks like: x = x 0 . The directed vector of the line is parallel to axis Oy as
− →
well and its projection on axis Ox equals to zero: s (0; n). In this case equation (6.8)
looks like: x−x 0 = y−y 0 . In an analogy, the canonical equation of a line, which is parallel
0 n
to axis Oy, looks like: x−x 0 = y−y 0 .
m 0

6.4.3. Equation of a line, that goes through a given point parallel to the given direction

Assume, there is a line l on a plane, that crosses axis Ox in point M 1 (fig. 6.10).
Angle α between axis Ox and line l is the smallest angle of turning around this point counter
clockwise until coinciding with line l. If a line coincides with axis Ox or is parallel to that axis,
then α = 0.
Let’s consider line l, which is not parallel to axis Oy . Its location is determined by angle α
and pointM 0 (x 0 ; y 0 ), which belongs to that line. Let’s take a single vector s = (cos α; cos β),
−→′
which forms the same angle α with axis Ox as the directed vector of that line. Obviously, cos β =
−→0
sin α, then s = (cos α; sin α). Having substituted m = cos α, n = sin α into equation (6.8),
we will get: x−x 0 = y−y 0 . Let’s solve the last equation relatively y−y 0 : y−y 0 = tg α·(x−x 0 ).
cos α sin α
Denote tg α = k, then
y − y 0 = k · (x − x 0 ). (6.9)

45