Page 43 - 4263

Equation of a line on a plane

A straight line can be written by equation F(r, φ) = 0, where (r; φ) are the polar coordinates
of any point, which belongs to that line.
Let’s consider another method of writing the equation of a straight line on a plane. Suppose,
two functions of the same parameter t are determined in system of coordinates Oxy : x = x(t),
y = y(t). Values of variables x and y for every value of parameter t are coordinates of some
point M. On changing t, variables x and y, generally speaking, are changing as well, so, point
M is said to go around the plane.

Definition 6.2. Equations x = x(t), y = y(t) are called parametric equations of
the trajectory of point M, argument t is called as varied parameter (for example, t
is time). ✓

Let’s consider examples of some lines.

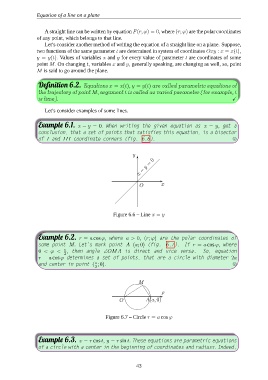
Example 6.1. x − y = 0. When writing the given equation as x = y, get a
conclusion, that a set of points that satisfies this equation, is a bisector
of I and III coordinate corners (fig. 6.6). ,

y
0
=
x − y

. . . . .
O x

Figure 6.6 – Line x = y

Example 6.2. r = a cos φ, where a > 0, (r; φ) are the polar coordinates of
some point M. Let’s mark point A (a; 0) (fig. 6.7). If r = a cos φ, where
0 < φ < π , then angle ∠OMA is direct and vice versa. So, equation
2
r = a cos φ determines a set of points, that are a circle with diameter 2a
a
and center in point ( ; 0). ,
2

M
ρ
. . . . .
O A(a, 0)

Figure 6.7 – Circle r = a cos φ

Example 6.3. x = τ cos t, y = τ sin t. These equations are parametric equations
of a circle with a center in the beginning of coordinates and radiusτ. Indeed,

43