Page 40 - 4263

Analytic geometry on a plane

Lecture 6. Analytic geometry on a plane

6.1. Polar system of coordinates

In Lecture 4 we discussed a rectangular system of coordinates. Let’s note that the system is
determined on a plane when a unit of calculating and two mutually perpendicular axes are given.
The point of crossing of these axes is called the beginning of coordinates, axes Ox and Oy are
coordinate axes (abscissa and ordinate). Every point is determined by means of two coordinates
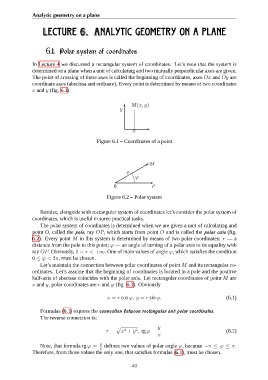
x and y (fig. 6.1).

M(x, y)
y

. . . .
x

Figure 6.1 – Coordinates of a point

M
ρ
φ
. . . . . .
0 ρ

Figure 6.2 – Polar system

Besides, alongside with rectangular system of coordinates let’s consider the polar system of
coordinates, which is useful in some practical tasks.
The polar system of coordinates is determined when we are given a unit of calculating and
point O, called the pole, ray OP, which starts from point O and is called the polar axis (fig.
6.2). Every point M in this system is determined by means of two polar coordinates: r — a
distance from the pole to this point; φ — an angle of turning of a polar axis to its equality with
ray OP. Obviously, 0 < r < +∞. One of main values of angle φ, which satisfies the condition
0 ≤ φ < 2π, must be chosen.
Let’s maintain the connection between polar coordinates of point M and its rectangular co-
ordinates. Let’s assume that the beginning of coordinates is located in a pole and the positive
half-axis of abscissa coincides with the polar axis. Let rectangular coordinates of point M are
x and y, polar coordinates are r and φ (fig. 6.3). Obviously

x = r cos φ, y = r sin φ. (6.1)

Formulas (6.1) express the connection between rectangular and polar coordinates.
The reverse connection is:
√ y
2
2
r = x + y , tg φ = . (6.2)
x
Note, that formula tg φ = y defines two values of polar angle φ, because −π ≤ φ ≤ π.
x
Therefore, from those values the only one, that satisfies formulas (6.1), must be chosen.

40