Page 48 - 4263

Analytic geometry on a plane

y

. . . . . . . 3
O x
y = 1
x + −2
−2 3

Figure 6.13 – Line x + y = 1
3 −2

6.4.7. Normal equation of a line

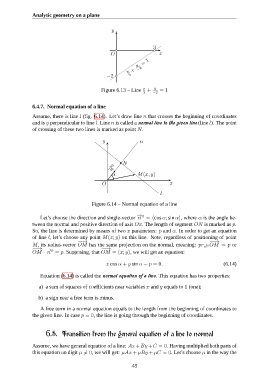
Assume, there is line l (fig. 6.14). Let’s draw line n that crosses the beginning of coordinates
and is y perpendicular to line l. Line n is called a normal line to the given line (line l). The point
of crossing of these two lines is marked as point N.

y n

N
− 0 n
→
α M(x, y)
. . . . . . . . . .
O x

l

Figure 6.14 – Normal equation of a line

− →0
Let’s choose the direction and single-vector n = (cos α; sin α), where α is the angle be-
tween the normal and positive direction of axis Ox. The length of segment ON is marked as p.
So, the line is determined by means of two x parameters: p and α. In order to get an equation
of line l, let’s choose any point M(x; y) on this line. Note, regardless of positioning of point
−−→ −−→
M, its radius-vector OM has the same projection on the normal, meaning: pr−→ 0OM = p or
n
−−→ −→0 −−→
OM · n = p. Supposing, that OM = (x; y), we will get an equation:
x cos α + y sin α − p = 0. (6.14)

Equation (6.14) is called the normal equation of a line. This equation has two properties:

a) a sum of squares of coefficients near variables x and y equals to 1 (one);

b) a sign near a free term is minus.

A free term in a normal equation equals to the length from the beginning of coordinates to
the given line. In case p = 0, the line is going through the beginning of coordinates.

6.5. Transition from the general equation of a line to normal

Assume, we have general equation of a line: Ax+By +C = 0. Having multiplied both parts of
this equation on digit µ ̸= 0, we will get: µAx + µBy + µC = 0. Let’s choose µ in the way the

48