Page 38 - 4263

Multiplication of Vectors

− → −→
− →
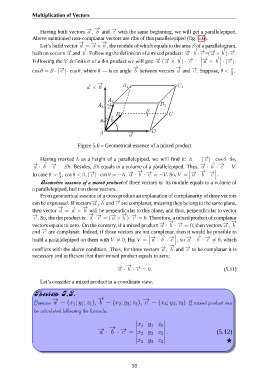
Having built vectors a , b and c with the same beginning, we will get a parallelepiped.
Above mentioned non-complanar vectors are ribs of this parallelepiped (fig. 5.6).
−→ −→ −→
Let’s build vector d = a × b , the module of which equals to the area S of a parallelogram,
−→ −→ − → −→ −→ − → − →
−→
built on vectors a and b . Following the definition of a mixed product: a · b · c =( a × b )· c .

−→
−→ −→ −→ −→ −→ −→
Following the V definition of a dot product we will get: a ( a × b ) · c = a × b · | c | ·
− → −→
−→
π
− →
cos θ = S · | c | · cos θ, where θ — is an angle b between vectors d and c . Suppose, θ < .
2
−→ −→ B 1 C 1
a × b
A 1
c
A 2 −→ D 1
S
B C
→
−
θ b
. . . . . . . . . . . . . . . .
A −→ D
a
Figure 5.6 – Geometrical essence of a mixed product

−→
Having marked h as a height of a parallelepiped, we will find it: h = | c | · cos θ. So,
−→ −→
−→ − → −→ −→
a · b · c = Sh. Besides, Sh equals to a volume of a parallelepiped. Thus, a · b · c = V.

−→ − → −→ −→
−→
−→
π
−→
In case θ > , cos θ < 0, | c | · cos θ = −h, a · b · c = −V. So, V = a · b · c .
2
Geometric essence of a mixed product of three vectors is: its module equals to a volume of
a parallelepiped, built on these vectors.
From geometrical essence of a cross product an explanation of complanarity of three vectors
−→
−→
−→
can be expressed. If vectors a , b and c are complanar, meaning they belong to the same plane,
−→ −→ −→
then vector d = a × b will be perpendicular to this plane, and thus, perpendicular to vector
−→ −→
−→ − → −→ − →
c . So, the dot product is: d · c = ( a × b )· c = 0. Therefore, a mixed product of complanar
−→ −→ −→ −→
− →
vectors equals to zero. On the contrary, if a mixed product a · b · c = 0, then vectors a , b
− →
and c are complanar. Indeed, if those vectors are not complanar, then it would be possible to
− → −→
−→
−→
−→
build a parallelepiped on them with V ̸= 0. But V = a · b · c , so a · b · c ̸= 0, which
−→
−→ −→
−→
conflicts with the above condition. Thus, for three vectors a , b and c to be complanar it is
necessary and sufficient that their mixed product equals to zero:
−→ −→ −→
a · b · c = 0. (5.11)
Let’s consider a mixed product in a coordinate view.
Theorem 5.3.
−→
Assume a = (x ; y ; z ), b = (x ; y ; z ), c = (x ; y ; z ). A mixed product can
−→
− →
3
3
3
1
2
1
2
2
1
be calculated following the formula:

x y z
1 1 1
−→
−→ −→
a · b · c = x 2 y 2 z . (5.12)

2

x 3 y 3 z 3 ⋆
38