Page 35 - 4263

Cross product of vectors

−→
c

. . . . . −→
−→ a
b φ

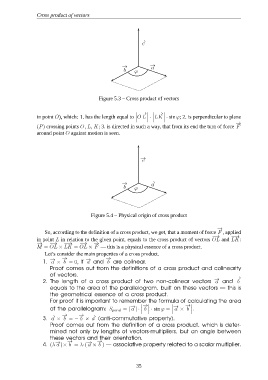
Figure 5.3 – Cross product of vectors

in point O), which: 1. has the length equal to O L · LK · sin φ; 2. is perpendicular to plane
−→ −→
−→
(P) crossing points O, L, K; 3. is directed in such a way, that from its end the turn of force F
around point O against motion is seen.

−→
c

. . . . . −→
−→ a
b φ

Figure 5.4 – Physical origin of cross product

−→
So, according to the definition of a cross product, we get, that a moment of force F , applied
−→ −−→
in point L in relation to the given point, equals to the cross product of vectors OL and LK:
−→ −→ −−→ −→ − →
M = OL × LK = OL × F — this is a physical essence of a cross product.
Let’s consider the main properties of a cross product.
−→
−→
−→
−→
1. a × b = 0, if a and b are colinear.
Proof comes out from the definitions of a cross product and colinearity
of vectors.
−→
−→
2. The length of a cross product of two non-colinear vectors a and b
equals to the area of the parallelogram, built on these vectors — this is
the geometrical essence of a cross product.
For proof it is important to remember the formula of calculating the area

−→
− →
−→
−→
of the parallelogram: S paral = | a | · b · sin φ = a × b .
−→ −→
−→
−→
3. a × b = − b × a (anti-commutative property).
Proof comes out from the definition of a cross product, which is deter-
mined not only by lengths of vectors-multipliers, but an angle between
these vectors and their orientation.
−→
−→
−→
−→
4. (λ a )× b = λ·( a × b ) — associative property related to a scalar multiplier.
35