Page 112 - 4624

1( θ and acts in the −∆u , direction; i.e.,
magnitude ∆ u ≈∆ ) r
θ
∆u θ = −∆ θu . Thus
r
∆u  ∆ θ 
u ɺ = lim θ =  lim  u
θ
t ∆ → 0 t ∆  t ∆ → 0 t ∆  r
ɺ
u ɺ θ = θu 2-24
r
Substituting this result and Eq. 2-19 into the above equation for
a, we can write the acceleration in component form as
a = a u r + a u 2-25
θ
r
θ
where
a =− rθ 2 ɺ 2-26
r ɺɺ
r
ɺɺ
a = rθ + 2rθ ɺ ɺ
θ
ɺɺ
)
2
The term θ = d θ / dt = d / dt (dθ / dt is called the angular
2
acceleration since it measures the change made in the angular velocity
2
during an instant of time. Units for this measurement are rad/s .

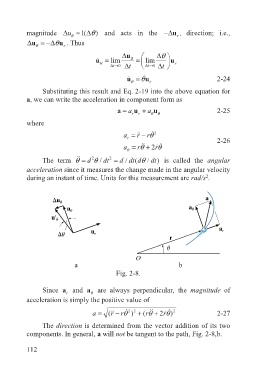
a b
Fig. 2-8.

Since a and a are always perpendicular, the magnitude of
r
θ
acceleration is simply the positive value of
ɺɺ
ɺ
−
ɺɺ
a = (rrθ 22 (rθ + ɺ ɺ ) 2-27
) +
2rθ
2
The direction is determined from the vector addition of its two
components. In general, a will not be tangent to the path, Fig. 2-8,b.
112