Page 114 - 4624

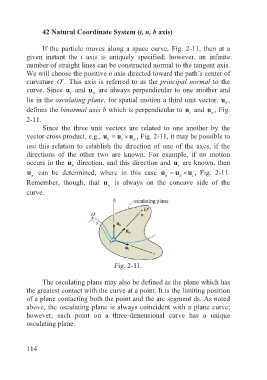
42 Natural Coordinate System (t, n, b axis)

If the particle moves along a space curve, Fig. 2-11, then at a
given instant the t axis is uniquely specified; however, an infinite
number of straight lines can be constructed normal to the tangent axis.
We will choose the positive n axis directed toward the path’s center of
curvature Oc. This axis is referred to as the principal normal to the
curve. Since u and u are always perpendicular to one another and
t
n
lie in the osculating plane, for spatial motion a third unit vector, u ,
b
defines the binormal axis b which is perpendicular to u and u , Fig.
t
n
2-11.
Since the three unit vectors are related to one another by the
vector cross product, e.g., u b u t uu , Fig. 2-11, it may be possible to
n
use this relation to establish the direction of one of the axes, if the
directions of the other two are known. For example, if no motion
occurs in the u direction, and this direction and u are known, then
t
b
u can be determined, where in this case u n u b uu , Fig. 2-11.
t
n
Remember, though, that u is always on the concave side of the
n
curve.

Fig. 2-11.

The osculating plane may also be defined as the plane which has
the greatest contact with the curve at a point. It is the limiting position
of a plane contacting both the point and the arc segment ds. As noted
above, the osculating plane is always coincident with a plane curve;
however, each point on a three-dimensional curve has a unique
osculating plane.

114