Page 113 - 4624

Cylindrical Coordinates. If the particle moves along a space
curve as shown in Fig. 2-9, then its location may be specified by the
three cylindrical coordinates r, θ, z. The z coordinate is identical to
that used for rectangular coordinates. Since the unit vector defining its
direction u is constant, the time derivatives of this vector are zero,
z
and therefore the position, velocity, and acceleration of the particle
can be written in terms of its cylindrical coordinates as follows:
r P = ru r + zu 2-28
z
ɺ
v = ru ɺ r + rθu θ + zu ɺ 2-29
z
ɺɺ
2rθ
a = ɺɺ 2 ɺ )u r + (rθ + ɺ ɺ )u θ + zu ɺɺ 2-30
(rrθ−
z

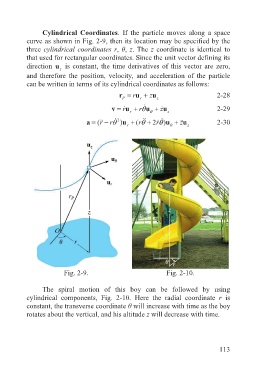
Fig. 2-9. Fig. 2-10.

The spiral motion of this boy can be followed by using
cylindrical components, Fig. 2-10. Here the radial coordinate r is
constant, the transverse coordinate θ will increase with time as the boy
rotates about the vertical, and his altitude z will decrease with time.

113