Page 110 - 4624

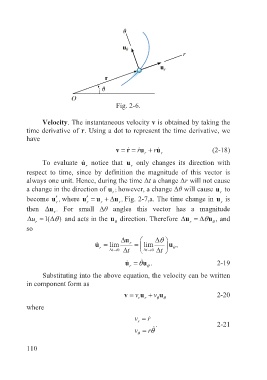
Fig. 2-6.

Velocity. The instantaneous velocity v is obtained by taking the
time derivative of r. Using a dot to represent the time derivative, we
have
v ==r ɺ ru ɺ r + ru ɺ r (2-18)

To evaluate u ɺ notice that u only changes its direction with
r
r
respect to time, since by definition the magnitude of this vector is
always one unit. Hence, during the time ∆t a change ∆r will not cause
a change in the direction of u ; however, a change ∆θ will cause u to
r
r
become ′ u , where ′ =u r u r +∆u , Fig. 2-7,a. The time change in u is
r
r
r
then ∆u . For small ∆θ angles this vector has a magnitude
r
∆ u ≈∆ ) θ r =∆ θu , and
1( θ and acts in the u direction. Therefore ∆u
θ
r
so
∆u  ∆ θ 
u ɺ r = lim 0 t ∆ r =   lim 0 t ∆   u ,
θ
t ∆ →
t ∆ →
ɺ
u ɺ r = θu . 2-19
θ
Substituting into the above equation, the velocity can be written
in component form as
v = v u r + v u 2-20
θ
r
θ
where
v = ɺ . 2-21
r
r
ɺ
v = rθ
θ
110