Page 115 - 4624

43 Motion of Particle: Natural Coordinate System

When the path along which a particle travels is known, then it is
often convenient to describe the motion using t, n, and b coordinate
axes which act tangent, normal and binormal to the path, respectively,
and at the instant considered have their origin located at the particle.
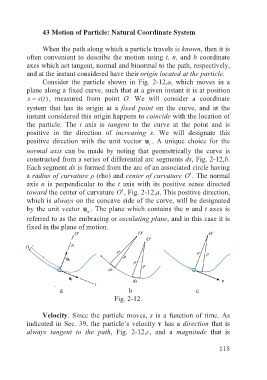
Consider the particle shown in Fig. 2-12,a, which moves in a
plane along a fixed curve, such that at a given instant it is at position
(), measured from point O. We will consider a coordinate
s st
system that has its origin at a fixed point on the curve, and at the
instant considered this origin happens to coincide with the location of
the particle. The t axis is tangent to the curve at the point and is
positive in the direction of increasing s. We will designate this
positive direction with the unit vector u . A unique choice for the
t
normal axis can be made by noting that geometrically the curve is
constructed from a series of differential arc segments ds, Fig. 2-12,b.
Each segment ds is formed from the arc of an associated circle having
a radius of curvature ȡ (rho) and center of curvature Oc. The normal
axis n is perpendicular to the t axis with its positive sense directed
toward the center of curvature Oc, Fig. 2-12,a. This positive direction,
which is always on the concave side of the curve, will be designated
by the unit vector u . The plane which contains the n and t axes is
n
referred to as the embracing or osculating plane, and in this case it is
fixed in the plane of motion.

a b c
Fig. 2-12.

Velocity. Since the particle moves, s is a function of time. As
indicated in Sec. 39, the particle’s velocity v has a direction that is
always tangent to the path, Fig. 2-12,c, and a magnitude that is

115