Page 106 - 4624

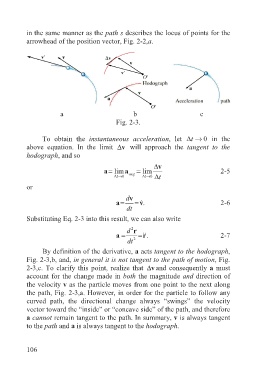
in the same manner as the path s describes the locus of points for the
arrowhead of the position vector, Fig. 2-2,a.

a b c
Fig. 2-3.

0
To obtain the instantaneous acceleration, let ∆→ in the
t
above equation. In the limit ∆v will approach the tangent to the
hodograph, and so
∆v
a = lima = lim 2-5
t ∆ → 0 avg t ∆→ 0 t ∆
or
dv
a = = . v ɺ 2-6
dt
Substituting Eq. 2-3 into this result, we can also write
d r
2
a = =r . ɺɺ 2-7
dt 2
By definition of the derivative, a acts tangent to the hodograph,
Fig. 2-3,b, and, in general it is not tangent to the path of motion, Fig.
2-3,c. To clarify this point, realize that ∆v and consequently a must
account for the change made in both the magnitude and direction of
the velocity v as the particle moves from one point to the next along
the path, Fig. 2-3,a. However, in order for the particle to follow any
curved path, the directional change always “swings” the velocity
vector toward the “inside” or “concave side” of the path, and therefore
a cannot remain tangent to the path. In summary, v is always tangent
to the path and a is always tangent to the hodograph.

106