Page 22 - 4498

Mg
p  p  ( exp ) h . (1.11.6)
0
RT
The derived equation (11.6) is a barometric formula that shows how the

pressure changes with altitude. This equation is derived assuming that the
temperature of the atmosphere is the same at every altitude (clearly not
always true). For this reason it is a model of an isothermal atmosphere.

According to the main equation of molecular-kinetic theory of ideal
gas pressure is equal to p  nkT and p  n 0 kT . If we substitute p and
0
p 0 values in the formula (11.6), we obtain the following:

Mg
n  n  ( exp ) h (1.11.7)
0
RT

n - the concentration of molecules at a height h

n - the concentration of molecules at a height h = 0.
0

Because M  m N andR  k  N
A
A
mgh
n  n  ( exp ) (1.11.8)
0
kT

or

n mgh
 ( exp ) (1.11.9)
n 0 kT

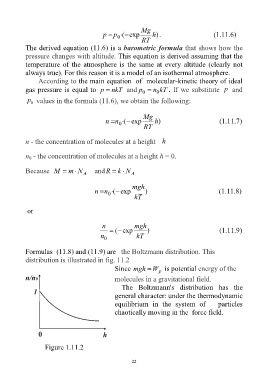
Formulas (11.8) and (11.9) are the Boltzmann distribution. This
distribution is illustrated in fig. 11.2

Since mgh  W is potential energy of the
p
molecules in a gravitational field.

The Boltzmann's distribution has the
general character: under the thermodynamic

equilibrium in the system of particles
chaotically moving in the force field.

Figure 1.11.2

22