Page 19 - 4498

substantially change the pressure exerted by them. Neither of those effects
are considered by the ideal gas model

1.10. Distribution of Molecular Velocities in Gases.

Maxwellian Distribution

Although molecules collide frequently with other molecules, and

although the velocity of any individual molecule undergoes sharp changes
but the average kinetic energy of the molecules is the same at a given
temperature. Some molecules are moving rapidly, and some slowly. Let us
consider the distribution of speeds for gas molecules

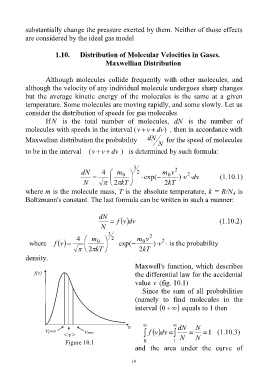
If N is the total number of molecules, dN is the number of
molecules with speeds in the interval (v  v  dv) , then in accordance with

Maxwelian distribution the probability dN for the speed of molecules
N
to be in the interval (v  v  dv ) is determined by such formula:

3 2
dN 4  m 0  2 m 0 v 2
   exp(   ) v  dv (1.10.1)
N   2 kT  2 kT
where m is the molecule mass, T is the absolute temperature, k = R/N is
A
Boltzmann's constant. The last formula can be written in such a manner:

dN
 f  dvv (1.10.2)
N
3 2
4  m  2 m v 2
where   vf  0  exp(  0  ) v  is the probability
  2 kT  2kT

density.
Maxwell's function, which describes
the differential law for the accidental

value v (fig. 10.1)
Since the sum of all probabilities
(namely to find molecules in the
interval  0   equals to 1 then

  dN N
 f  dvv     1 (1.10.3)

Figure 10.1 0 1 N N
and the area under the curve of

19