Page 59 - 4263

Hyperbola

let’s introduce the definition of directrices for that lines.

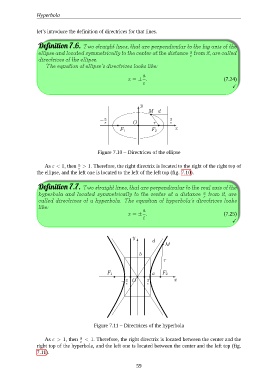
Definition 7.6. Two straight lines, that are perpendicular to the big axis of the
ellipse and located symmetrically to the center at the distance a ε from it, are called
directrices of the ellipse.
The equation of ellipse’s directrices looks like:

a
x = ± . (7.24)
ε
✓

y
M d
a a
−
ε O . . . . . . . . . . . τ ε
x
F 1 F 2

Figure 7.10 – Directrices of the ellipse

As ε < 1, then a > 1. Therefore, the right directrix is located to the right of the right top of
ε
the ellipse, and the left one is located to the left of the left top (fig. 7.10).

Definition 7.7. Two straight lines, that are perpendicular to the real axis of the
hyperbola and located symmetrically to the center at a distance a from it, are
ε
called directrices of a hyperbola. The equation of hyperbola’s directrices looks
like:
a
x = ± . (7.25)
ε
✓

y
d
M

b
τ

F 1 a F 2
. . . . . . . . . . . . .
a O a x
−
ε ε

Figure 7.11 – Directrices of the hyperbola

As ε > 1, then a < 1. Therefore, the right directrix is located between the center and the
ε
right top of the hyperbola, and the left one is located between the center and the left top (fig.
7.11).

59