Page 61 - 4263

Parabola

then for point M : ε > d, which means this point doesn’t belong to the parabola. Using formula
(7.26), we will get:

√
p p
2
2
(x − ) + y = x + . (7.27)
2 2
Equation (7.27) is the parabola’s equation. Having squared both parts of this equation, we
will make it look simplier:
p 2 p 2
2
2
2
2
x − px + + y = x + px + or y = 2px. (7.28)
4 4
In order to check the equivalence of equations (7.27) and (7.28), it is enough to prove, that
for any point M(x; y), coordinates of which satisfy equation (7.28), where x ≥ 0, equation
(7.27) takes place as well. Equation (7.28) is called the canonical equation of the parabola. It is
clear, that the parabola is the line of the second order.

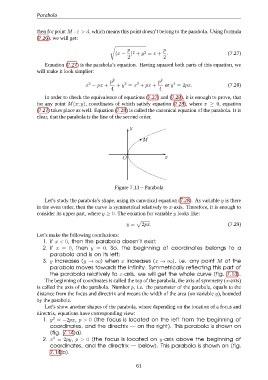
y

M

. . . . .
O x

Figure 7.13 – Parabola

Let’s study the parabola’s shape, using its canonical equation (7.28). As variable y is there
in the even order, then the curve is symmetrical relatively to x-axis. Therefore, it is enough to
consider its upper part, where y ≥ 0. The equation for variable y looks like:

√
y = 2px. (7.29)
Let’s make the following conclusions:
1. if x < 0, then the parabola doesn’t exist;
2. if x = 0, then y = 0. So, the beginning of coordinates belongs to a
parabola and is on its left;
3. y increases (y → ∞) when x increases (x → ∞), i.e. any point M of the
parabola moves towards the infinity. Symmetrically reflecting this part of
the parabola relatively to x-axis, we will get the whole curve (fig. 7.13).
The beginning of coordinates is called the top of the parabola, the axis of symmetry (x-axis)
is called the axis of the parabola. Number p, i.e. the parameter of the parabola, equals to the
distance from the focus and directrix and means the width of the area (on variable y), bounded
by the parabola.
Let’s show another shapes of the parabola, where depending on the location of a focus and
directrix, equations have corresponding view:
2
1. y = −2px, p > 0 (the focus is located on the left from the beginning of
coordinates, and the directrix — on the right). This parabola is shown on
(fig. 7.14)a).
2. x = 2py, p > 0 (the focus is located on y-axis above the beginning of
2
coordinates, and the directrix — below). This parabola is shown on (fig.
7.14)b).

61