Page 64 - 4263

Analytic geometry in space

y y ′
y ′′

x ′′

. . . . . . . . . . α
O ′ x ′

O x

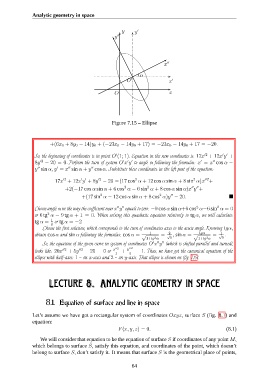
Figure 7.15 – Ellipse

+(6x 0 + 8y 0 − 14)y 0 + (−23x 0 − 14y 0 + 17) = −23x 0 − 14y 0 + 17 = −20.

′2
′
So, the beginning of coordinates is in point O (1; 1). Equation in the new coordinates is: 17x + 12x y +
′ ′
′2 ′ ′ ′ ′ ′′
8y − 20 = 0. Perform the turn of system O x y to angle α following the formulas: x = x cos α −
y sin α, y = x sin α + y cos α. Substitute these coordinates in the left part of the equation:
′
′′
′′
′′
2
′2
2
′′2
′2
′ ′
17x + 12x y + 8y − 20 =(17 cos α + 12 cos α sin α + 8 sin α)x +
2
2
′′ ′′
+2(−17 cos α sin α + 6 cos α − 6 sin α + 8 cos α sin α)x y +
2
2
′′
+(17 sin α − 12 cos α sin α + 8 cos α)y − 20.
2
2
Choose angle α in the way the coefficient near x y equals to zero: −9 cos α sin α+6 cos α−6 sin α = 0
′′ ′′
or 6 tg α − 9 tg α + 1 = 0. When solving this quadratic equation relatively to tg α, we will calculate:
2
tg α = 1 or tg α = −2.
2
Choose the first solution, which corresponds to the turn of coordinates axes to the acute angle. Knowing tgα,
1 2 tgα 1
obtain cos α and sin α following the formulas: cos α = √ = √ , sin α = √ = √ .
2
2
1+tg α 5 1+tg α 5
So, the equation of the given curve in system of coordinates O x y (which is shifted parallel and turned),
′ ′′ ′′
looks like: 20x ′′2 + 5y ′′2 − 20 = 0 or x ′′2 + y ′′2 = 1. Thus, we have got the canonical equation of the
4
1
ellipse with half-axes: 1 — on x-axis and 2 — on y-axis. That ellipse is shown on (fig. 7.15).
Lecture 8. Analytic geometry in space
8.1. Equation of surface and line in space
Let’s assume we have got a rectangular system of coordinates Oxyz, surface S (fig. 8.1) and
equation:
F(x, y, z) = 0. (8.1)
We will consider that equation to be the equation of surface S if coordinates of any point M,
which belongs to surface S, satisfy this equation, and coordinates of the point, which doesn’t
belong to surface S, don’t satisfy it. It means that surface S is the geometrical place of points,

64