Page 57 - 4263

Hyperbola

y

B(a, b)

. . . . . .
O A(a, 0) x

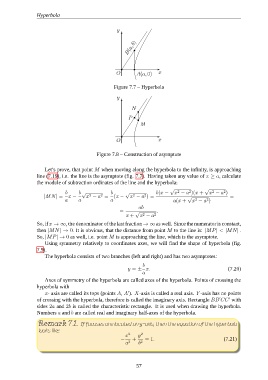
Figure 7.7 – Hyperbola
y

N
P
M

. . . . . . .
O x

Figure 7.8 – Construction of asymptote

Let’s prove, that point M when moving along the hyperbola to the infinity, is approaching
line (7.19), i.e. the line is the asymptote (fig. 7.7). Having taken any value of x ≥ a, calculate
the module of subtraction ordinates of the line and the hyperbola:
√ √
2
2
2
2
b b √ b √ b(x − x − a )(x + x − a )
2
2
2
2
|MN| = x − x − a = (x − x − a ) = √ =
a a a a(x + x − a )
2
2
ab
= √ .
x + x − a 2
2
So, if x → ∞, the denominator of the last fraction → ∞ as well. Since the numerator is constant,
then |MN| → 0. It is obvious, that the distance from point M to the line is: |MP| < |MN| .
So, |MP| → 0 as well, i.e. point M is approaching the line, which is the asymptote.
Using symmetry relatively to coordinates axes, we will find the shape of hyperbola (fig.
7.9).
The hyperbola consists of two branches (left and right) and has two asymptotes:
b
y = ± x. (7.20)
a
Axes of symmetry of the hyperbola are called axes of the hyperbola. Points of crossing the
hyperbola with
x- axis are called its tops (points A, A ). X-axis is called a real axis. Y -axis has no points
′
of crossing with the hyperbola, therefore is called the imaginary axis. Rectangle BB CC with
′
′
sides 2a and 2b is called the characteristic rectangle. It is used when drawing the hyperbola.
Numbers a and b are called real and imaginary half-axes of the hyperbola.
Remark 7.1. If focuses are located on y-axis, then the equation of the hyperbola
looks like:
x 2 y 2
− + = 1. (7.21)
a 2 b 2

57