Page 62 - 4263

Lines of the second order

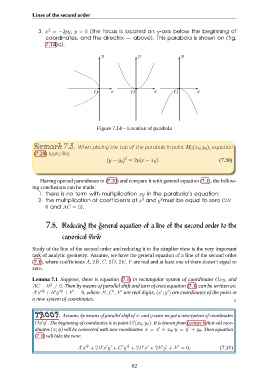
3. x = −2py, p > 0 (the focus is located on y-axis below the beginning of
2
coordinates, and the directrix — above). This parabola is shown on (fig.
7.14)c).

y y y

. . . . . . . . . . . .
O x O x O x

Figure 7.14 – Location of parabola

Remark 7.3. When placing the top of the parabola in point M 0 (x 0 ; y 0 ), equation
(7.28) looks like:
2
(y − y 0 ) = 2p(x − x 0 ). (7.30)

Having opened parentheses in (7.30) and compare it with general equation (7.1), the follow-
ing conclusions can be made:
1. there is no term with multiplication xy in the parabola’s equation;
2
2
2. the multiplication of coefficients at x and y must be equal to zero (2B =
0 and AC = 0).

7.5. Reducing the general equation of a line of the second order to the

canonical view

Study of the line of the second order and reducing it to the simplier view is the very important
task of analytic geometry. Assume, we have the general equation of a line of the second order
(7.1), where coefficients A, 2B, C, 2D, 2E, F are real and at least one of them doesn’t equal to
zero.

Lemma 7.1 Suppose, there is equation (7.1) in rectangular system of coordinates Oxy, and
2
AC −B ̸= 0. Then by means of parallel shift and turn of axes equation (7.1) can be written as:
′ ′′2
′ ′′2
A x + B y + F = 0, where A , C , F are real digits, (x ; y ) are coordinates of the point in
′
′
′
′
′
′
a new system of coordinates. 2
PROOF. Assume, by means of parallel shift of x- and y-axes we get a new system of coordinates
Ox y . The beginning of coordinates is in point O (x 0 ; y 0 ). It is known from Lecture 6 that old coor-
′ ′
′
dinates (x; y) will be connected with new coordinates: x = x + x 0 ; y = y + y 0 . Then equation
′
′
(7.1) will take the view:
′ ′2
′ ′2
′
′ ′ ′
′ ′
′ ′
A x + 2B x y + C y + 2D x + 2E y + F = 0, (7.31)
62