Page 58 - 4263

Lines of the second order

y

B ′ b B

F 1 A ′ . . . . . . . . . . . . . . A a F 2
O x

C ′ C

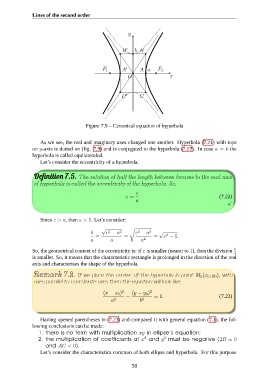
Figure 7.9 – Canonical equation of hyperbola

As we see, the real and imaginary axes changed one another. Hyperbola (7.21) with tops
on y-axis is dotted on (fig. 7.9) and is conjugated to the hyperbola (7.17). In case a = b the
hyperbola is called equilateraled.
Let’s consider the eccentricity of a hyperbola.

Definition 7.5. The relation of half the length between focuses to the real axis
of hyperbola is called the eccentricity of the hyperbola. So,

c
ε = . (7.22)
a
✓

Since c > a, then ε > 1. Let’s consider:

√ √
2
b c − a 2 c − a 2 √
2
= = = ε − 1.
2
a a a 2
So, the geometrical content of the eccentricity is: if ε is smaller (nearer to 1), then the division b
a
is smaller. So, it means that the characteristic rectangle is prolonged in the direction of the real
axis and characterises the shape of the hyperbola.

Remark 7.2. If we place the center of the hyperbola in point M 0 (x 0 ; y 0 ), with
axes parallel to coordinate axes then the equation will look like:

(x − x 0 ) 2 (y − y 0 ) 2
− = 1. (7.23)
a 2 b 2

Having opened parentheses in (7.23) and compared it with general equation (7.1), the fol-
lowing conclusions can be made:
1. there is no term with multiplication xy in ellipse’s equation;
2
2
2. the multiplication of coefficients at x and y must be negative (2B = 0
and AC < 0).
Let’s consider the characteristics common of both ellipse and hyperbola. For this purpose

58