Page 54 - 4263

Lines of the second order

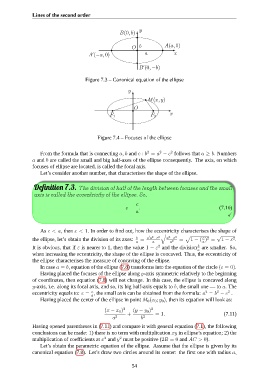
y
B(0, b)

O . . . . . . . . . . b A(a, 0)

A (−a, 0) a x
′
′
B (0, −b)

Figure 7.3 – Canonical equation of the ellipse
y

M(x, y)

. . . . . . . O
x
F 1 F 2

Figure 7.4 – Focuses of the ellipse

2
2
2
From the formula that is connecting a, b and c : b = a − c follows that a ≥ b. Numbers
a and b are called the small and big half-axes of the ellipse consequently. The axis, on which
focuses of ellipse are located, is called the focal axis.
Let’s consider another number, that characterises the shape of the ellipse.

Definition 7.3. The division of half of the length between focuses and the small
axis is called the eccentricity of the ellipse. So,

c
ε = . (7.10)
a
✓

As c < a, then ε < 1. In order to find out, how the eccentricity characterises the shape of
√
√ 2 2 2 2 √ √
2
c 2
the ellipse, let’s obtain the division of its axes: b = a −c a −c = 1 − ( ) = 1 − ε .
a a a 2 a
b
2
It is obvious, that if ε is nearer to 1, then the value 1 − ε and the division are smaller. So,
a
when increasing the eccentricity, the shape of the ellipse is concaved. Thus, the eccentricity of
the ellipse characterises the measure of concaving of the ellipse.
In case a = b, equation of the ellipse (7.8) transforms into the equation of the circle (ε = 0).
Having placed the focuses of the ellipse along y-axis symmetric relatively to the beginning
of coordinates, then equation (7.8) will not change. In this case, the ellipse is concaved along
y-axis, i.e. along its focal axis, and so, its big half-axis equals to b, the small one — to a. The
2
c
2
2
eccentricity equals to: ε = , the small axis can be obtained from the formula: a = b − c .
b
Having placed the center of the ellipse in point M 0 (x 0 ; y 0 ), then its equation will look as:
(x − x 0 ) 2 (y − y 0 ) 2
+ = 1. (7.11)
a 2 b 2
Having opened parentheses in (7.11) and compare it with general equation (7.1), the following
conclusions can be made: 1) there is no term with multiplication xy in ellipse’s equation; 2) the
2
2
multiplication of coefficients at x and y must be positive (2B = 0 and AC > 0).
Let’s obtain the parametric equation of the ellipse. Assume that the ellipse is given by its
canonical equation (7.8). Let’s draw two circles around its center: the first one with radius a,

54