Page 55 - 4263

Hyperbola

y

b P

Q M

. . . . . . . . . . . . α Q 1 P 1
O a x

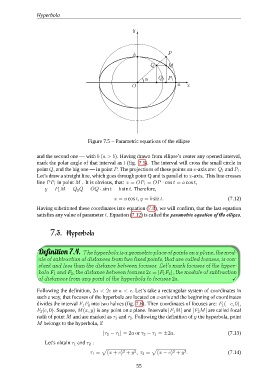
Figure 7.5 – Parametric equations of the ellipse

and the second one — with b (a > b). Having drawn from ellipse’s center any opened interval,
mark the polar angle of that interval as t (fig. 7.5). The interval will cross the small circle in
point Q, and the big one — in point P. The projections of these points on x-axis are: Q 1 and P 1 .
Let’s draw a straight line, which goes through point Q and is parallel to x-axis. This line crosses
line PP 1 in point M . It is obvious, that: x = OP 1 = OP · cos t = a cos t,
y = P 1 M = Q 1 Q = OQ · sin t = b sin t. Therefore,
x = a cos t, y = b sin t. (7.12)

Having substituted these coordinates into equation (7.8), we will confirm, that the last equation
satisfies any value of parameter t. Equation (7.12) is called the parametric equation of the ellipse.

7.3. Hyperbola

Definition 7.4. The hyperbola is a geometric place of points on a plane, the mod-
ule of subtraction of distances from two fixed points, that are called focuses, is con-
stant and less than the distance between focuses. Let’s mark focuses of the hyper-
bola F 1 and F 2 , the distance between focuses 2c = |F 1 F 2 | , the module of subtraction

of distances from any point of the hyperbola to focuses 2a. ✓

Following the definition, 2a < 2c or a < c. Let’s take a rectangular system of coordinates in
such a way, that focuses of the hyperbola are located on x-axis and the beginning of coordinates
divides the interval F 1 F 2 into two halves (fig. 7.6). Then coordinates of focuses are: F 1 (−c, 0),
F 2 (c, 0). Suppose, M(x, y) is any point on a plane. Intervals |F 1 M| and |F 2 M| are called focal
radii of point M and are marked as τ 1 and τ 2 . Following the definition of y the hyperbola, point
M belongs to the hyperbola, if

|τ 2 − τ 1 | = 2a or τ 2 − τ 1 = ±2a. (7.13)
Let’s obtain τ 1 and τ 2 :
√ √
2
2
2
2
τ 1 = (x + c) + y , τ 2 = (x − c) + y . (7.14)
55