Page 60 - 4263

Lines of the second order

Let’s formulate the theorem, which expresses the common of both ellipse and hyperbola.

Theorem 7.1.
Let’s assume that r is the focal radius of any point M of the ellipse (or hyperbola), d is the

distance from this point to the corresponding directrix. Then division r is constant and equals
d
to the eccentricity of the ellipse (hyperbola). ⋆

PROOF. ( for the ellipse). Let’s choose the right focus F 2 (c; 0) and the right directrix. Suppose
√ c
a
2
2
M(x; y) is any point on the line. Distance d = − x, r = τ 2 = (x − c) + y = a − x.
ε a
c
Having substituted by ε, we will get: r = a − εx. Then
a
r a − εx (a − εx)ε
= = = ε. (7.26)
d a − x a − εx
ε
The proof for the hyperbola is the same. 2
By means of this property the common definition of the ellipse and hyperbola can be ex-
pressed like this: the geometrical place of points, for which the relation of distances to the focus
and corresponding directrix is constant, equals to ε, is called an ellipse (if ε < 1), and a hyper-
bola (if ε > 1). In case ε = 1, those geometrical points are a parabola.

7.4. Parabola

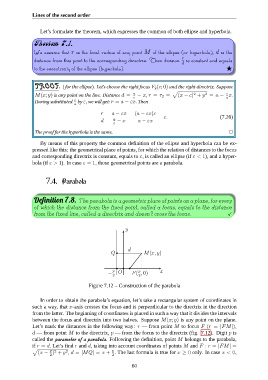
Definition 7.8. The parabola is a geometric place of points on a plane, for every
of which the distance from the fixed point, called a focus, equals to the distance
from the fixed line, called a directrix and doesn’t cross the focus. ✓

y

d
Q M(x, y)

. . . . . . . . .
p O p x
− F( , 0)
2 2
Figure 7.12 – Construction of the parabola

In order to obtain the parabola’s equation, let’s take a rectangular system of coordinates in
such a way, that x-axis crosses the focus and is perpendicular to the directrix in the direction
from the latter. The beginning of coordinates is placed in such a way that it divides the intervals
between the focus and directrix into two halves. Suppose M(x; y) is any point on the plane.
Let’s mark the distances in the following way: r — from point M to focus F (r = |FM|),
d — from point M to the directrix, p — from the focus to the directrix (fig. 7.12). Digit p is
called the parameter of a parabola. Following the definition, point M belongs to the parabola,
if r = d. Let’s find r and d, taking into account coordinates of points M and F : r = |FM| =
√ p 2 p
2
(x − ) + y , d = |MQ| = x + . The last formula is true for x ≥ 0 only. In case x < 0,
2 2
60