Page 51 - 4263

Solution. Obviously, k 1 = 2, k 2 = 3. Using formula (6.19), we will get: tg φ = −3−2 = 1. Thus,
1+(−3)·2
π
the acute angle between given lines is: φ = , the obtuse angle equals to: π − φ = 3π .
4 4

Lecture 7. Lines of the second order

Such curves as circle, ellipse, hyperbola and parabola belong to lines of the second order.
All these lines are described by equations of the second order relatively to variables x and y. In
general, these equations look like:

2
2
Ax + 2Bxy + Cy + 2Dx + 2Ey + F = 0, (7.1)
where coefficients A, 2B, C, 2D, 2E, F are real and at least one of them doesn’t equal to zero.
Let’s characterise every line.

7.1. Circle

Definition 7.1. A circle is a geometric place of points on a plane, equally dis-
tanced from the fixed point, which is called a center. According to the definition,
the distance from any point of a circle to its center is constant, called as the radius
and is written R. ✓

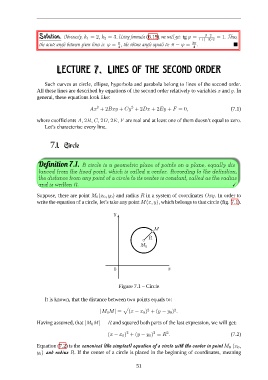
Suppose, there are point M 0 (x 0 , y 0 ) and radius R in a system of coordinates Oxy. In order to
write the equation of a circle, let’s take any point M(x, y), which belongs to that circle (fig. 7.1).

y

M
R

M 0

. . . . . . .
0 x

Figure 7.1 – Circle

It is known, that the distance between two points equals to:

√
2
2
|M 0 M| = (x − x 0 ) + (y − y 0 ) .
Having assumed, that |M 0 M| = R and squared both parts of the last expression, we will get:

2
2
2
(x − x 0 ) + (y − y 0 ) = R . (7.2)
Equation (7.2) is the canonical (the simplest) equation of a circle with the center in point M 0 (x 0 ,
y 0 ) and radius R. If the center of a circle is placed in the beginning of coordinates, meaning

51