Page 105 - 6099

Maximum Principle. Let D be a connected bounded open set (in either two- or
three-dimensional space). Let either u(x, y) or u(x, y, z) be a harmonic function in D
that is continuous on D = D ∪ (boundaryD). Then the maximum and the minimum
values of u are attained on boundary D and nowhere inside (unless u ≡ constant).
In other words, a harmonic function is its biggest somewhere on the boundary and its
smallest somewhere else on the boundary.

10.2 Polar coordinates

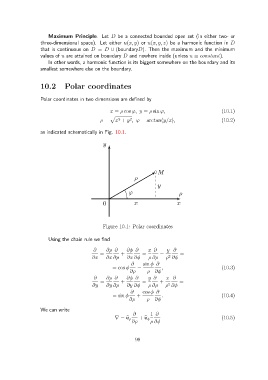
Polar coordinates in two dimensions are defined by

x = ρ cos ϕ, y = ρ sin ϕ, (10.1)
p
2
2
ρ = x + y , ϕ = arctan(y/x), (10.2)
as indicated schematically in Fig. 10.1.

y

M
ρ
y
ϕ ρ

0 x x

Figure 10.1: Polar coordinates

Using the chain rule we find

∂ ∂ρ ∂ ∂φ ∂ x ∂ y ∂
= + = − =
∂x ∂x ∂ρ ∂x ∂φ ρ ∂ρ ρ ∂φ
2
∂ sin φ ∂
= cos φ − , (10.3)
∂ρ ρ ∂φ
∂ ∂ρ ∂ ∂φ ∂ y ∂ x ∂
= + = + =
2
∂y ∂y ∂ρ ∂y ∂φ ρ ∂ρ ρ ∂φ
∂ cos φ ∂
= sin φ + . (10.4)
∂ρ ρ ∂φ

We can write
∂ 1 ∂
∇ = b e ρ + b e φ (10.5)
∂ρ ρ ∂φ

98