Page 107 - 6099

δρ

ρδϕ

ρ + δρ

ρ
ϕ

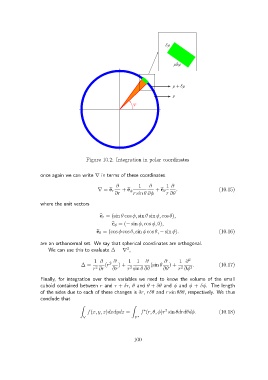
Figure 10.2: Integration in polar coordinates

once again we can write ∇ in terms of these coordinates.

∂ 1 ∂ 1 ∂
∇ = b e r + b e φ + b e θ . (10.15)
∂r r sin θ ∂φ r ∂θ

where the unit vectors

b e r = (sin θ cos φ, sin θ sin φ, cos θ),
b e φ = (− sin φ, cos φ, 0),

b e θ = (cos φ cos θ, sin φ cos θ, − sin φ). (10.16)

are an orthonormal set. We say that spherical coordinates are orthogonal.
2
We can use this to evaluate ∆ = ∇ ,

1 ∂ ∂ 1 1 ∂ ∂ 1 ∂ 2
∆ = (r 2 ) + (sin θ ) + . (10.17)
2
2
2
r ∂r ∂r r sin θ ∂θ ∂θ r ∂φ 2
Finally, for integration over these variables we need to know the volume of the small
cuboid contained between r and r + δr, θ and θ + δθ and φ and φ + δφ. The length
of the sides due to each of these changes is δr, rδθ and r sin θδθ, respectively. We thus
conclude that
Z Z
∗
2
f(x, y, z)dxdydz = f (r, θ, φ)r sin θdrdθdφ. (10.18)
V V ∗

100