Page 100 - 6099

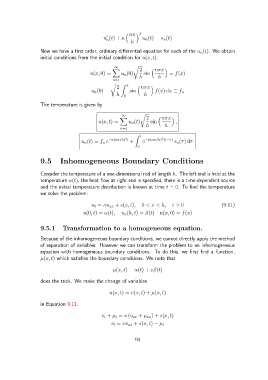
nπ 2
0
u (t) + κ u n (t) = s n (t)
n
h
Now we have a first order, ordinary differential equation for each of the u n (t). We obtain
initial conditions from the initial condition for u(x, t).

∞ r
X 2 nπx
u(x, 0) = u n (0) sin = f(x)
h h
n=1
r Z h
2 nπx
u n (0) = sin f(x) dx ≡ f n
h 0 h
The temperature is given by

∞ r
X 2 nπx
u(x, t) = u n (t) sin ,
h h
n=1
t
Z
2
2
e −κ(nπ/h) t + e −κ(nπ/h) (t−τ) s n (τ) dτ.
u n (t) = f n
0
9.5 Inhomogeneous Boundary Conditions

Consider the temperature of a one-dimensional rod of length h. The left end is held at the
temperature α(t), the heat flow at right end is specified, there is a time-dependent source
and the initial temperature distribution is known at time t = 0. To find the temperature
we solve the problem:

u t = κu xx + s(x, t), 0 < x < h, t > 0 (9.11)
u(0, t) = α(t), u x (h, t) = β(t) u(x, 0) = f(x)

9.5.1 Transformation to a homogeneous equation.

Because of the inhomogeneous boundary conditions, we cannot directly apply the method
of separation of variables. However we can transform the problem to an inhomogeneous
equation with homogeneous boundary conditions. To do this, we first find a function,
µ(x, t) which satisfies the boundary conditions. We note that

µ(x, t) = α(t) + xβ(t)

does the trick. We make the change of variables

u(x, t) = v(x, t) + µ(x, t)

in Equation 9.11.

v t + µ t = κ (v xx + µ xx ) + s(x, t)

v t = κv xx + s(x, t) − µ t

93