Page 108 - 6099

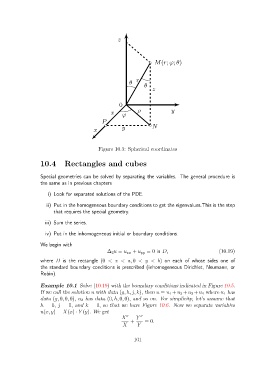
z

M(r; ϕ; θ)

r
θ
θ
z

0
x ϕ ρ y

P
x y N

Figure 10.3: Spherical coordinates

10.4 Rectangles and cubes

Special geometries can be solved by separating the variables. The general procedure is
the same as in previous chapters

i) Look for separated solutions of the PDE.

ii) Put in the homogeneous boundary conditions to get the eigenvalues.This is the step
that requires the special geometry.

iii) Sum the series.

iv) Put in the inhomogeneous initial or boundary conditions.

We begin with
∆ 2 u = u xx + u yy = 0 in D, (10.19)

where D is the rectangle (0 < x < a, 0 < y < b) on each of whose sides one of
the standard boundary conditions is prescribed (inhomogeneous Dirichlet, Neumann, or
Robin).

Example 10.1 Solve (10.19) with the boundary conditions indicated in Figure 10.5.
If we call the solution u with data (g, h, j, k), then u = u 1 +u 2 +u 3 +u 4 where u 1 has
data (g, 0, 0, 0), u 2 has data (0, h, 0, 0), and so on. For simplicity, let’s assume that
h = 0, j = 0, and k = 0, so that we have Figure 10.6. Now we separate variables
u(x, y) = X(x) · Y (y). We get
X 00 Y 00
+ = 0.
X Y

101