Page 81 - 4811

Application of Laplace Transform

simple example of an initial value problem for a 2 × 2 system.
. We illustrate with a few examples.

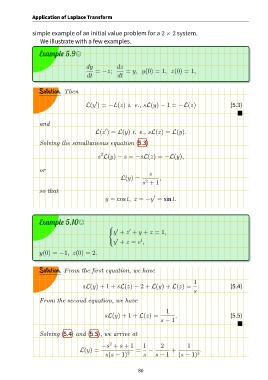
Example 5.9,

dy dz
= −z; = y, y(0) = 1, z(0) = 1,
dt dt
. . . . .
.

Solution. Then

L(y ) = −L(z) i. e., sL(y) − 1 = −L(z) (5.3)
′

and
′
L(z ) = L(y) i. e., sL(z) = L(y).
Solving the simultaneous equation (5.3)

2
s L(y) − s = −sL(z) = −L(y),

or
s
L(y) = ,
2
s + 1
so that
′
y = cos t, z = −y = sin t.
.
. . . .

Example 5.10,
{
y + z + y + z = 1,
′
′
t
′
y + z = e ,
y(0) = −1, z(0) = 2.
.
. . . . .
Solution. From the first equation, we have

1
sL(y) + 1 + sL(z) − 2 + L(y) + L(z) = . (5.4)
s
From the second equation, we have

1
sL(y) + 1 + L(z) = . (5.5)
s − 1

Solving (5.4) and (5.5), we arrive at

2
−s + s + 1 1 2 1
L(y) = = − + .
. . . . s(s − 1) 2 s s − 1 (s − 1) 2

80