Page 76 - 4811

General Solutions
.

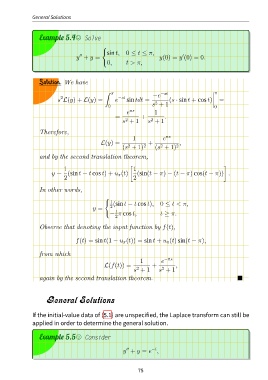
Example 5.4, Solve

{
sin t, 0 ≤ t ≤ π,
′′
′
y + y = y(0) = y (0) = 0.
0, t > π,
. . . . .
.
Solution. We have

π −e −st
∫ π
2
s L(y) + L(y) = e −st sin tdt = (s · sin t + cos t) =
2
0 s + 1 0
e πs 1
= + .
2
2
s + 1 s + 1
Therefore,
1 e πs
L(y) = + ,
2
2
(s + 1) 2 (s + 1) 2
and by the second translation theorem,

[ ]
1 1
y = (sin t − t cos t) + u (t) (sin(t − π) − (t − π) cos(t − π)) .
π
2 2
In other words,

{
1 (sin t − t cos t), 0 ≤ t < π,
y = 2
1
− π cos t, t ≥ π.
2
Observe that denoting the input function by f(t),

f(t) = sin t(1 − u (t)) = sin t + u (t) sin(t − π),
π
π
from which
1 e −πs
L(f(t)) = + ,
2
2
s + 1 s + 1
. . . . again by the second translation theorem.

General Solutions

If the initial-value data of (5.1) are unspecified, the Laplace transform can still be
.
applied in order to determine the general solution.

Example 5.5, Consider

−t
′′
y + y = e ,
. . . . .

75