Page 77 - 4811

Application of Laplace Transform
.

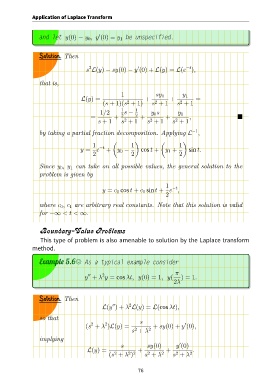
and let y(0) = y , y (0) = y be unspecified.
′
1
0
. . . . .
.
Solution. Then
2
−t
′
s L(y) − sy(0) − y (0) + L(y) = L(e ),
that is,

1 sy 0 y 1
L(y) = + + =
2
2
2
(s + 1)(s + 1) s + 1 s + 1
1 1
1/2 s − y s y 1
0
= + 2 2 + + ,
2
2
2
s + 1 s + 1 s + 1 s + 1
−1
by taking a partial fraction decomposition. Applying L ,
( ) ( )
1 1 1
y = e −t + y − cos t + y + sin t.
0
1
2 2 2
Since y , y can take on all possible values, the general solution to the
1
0
problem is given by
1
−t
y = c cos t + c sin t + e ,
0
1
2
where c , c are arbitrary real constants. Note that this solution is valid
0
1
for −∞ < t < ∞.
. . . .
Boundary-Value Problems

This type of problem is also amenable to solution by the Laplace transform
.
method.

Example 5.6, As a typical example consider

π
2
′′
y + λ y = cos λt, y(0) = 1, y( ) = 1.
2λ
. . . . .
.
Solution. Then
2
′′
L(y ) + λ L(y) = L(cos λt),
so that s
2
2
′
(s + λ )L(y) = + sy(0) + y (0),
2
s + λ 2
implying
s sy(0) y (0)
′
L(y) = + + .
2 2
2
2
2
. . . . (s + λ ) s + λ 2 s + λ 2
76