Page 34 - 4811

Partial Fractions

n
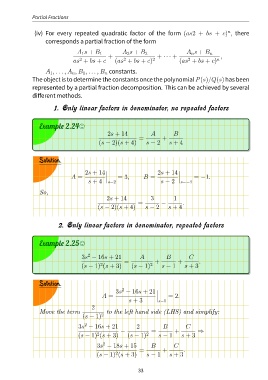
(iv) For every repeated quadratic factor of the form (as2 + bs + c) , there
corresponds a partial fraction of the form

A s + B 1 A s + B 2 A s + B n
2
1
n
+ + · · · + ,
2
2
2
as + bs + c (as + bs + c) 2 (as + bs + c) n
A , . . . , A , B , . . . , B constants.
1
1
n
n
The object is to determine the constants once the polynomial P(s)/Q(s) has been
represented by a partial fraction decomposition. This can be achieved by several
different methods.
1. Only linear factors in denominator, no repeated factors
.

Example 2.24,
2s + 14 A B
= +
(s − 2)(s + 4) s − 2 s + 4
. . . . .
.
Solution.

A = 2s + 14 = 3, B = 2s + 14 = −1.

s + 4 s=2 s − 2 s=−4
So,
2s + 14 3 1
= − .
(s − 2)(s + 4) s − 2 s + 4
. . . .
2. Only linear factors in denominator, repeated factors
.

Example 2.25,

2
3s − 16s + 21 A B C
= + + .
2
(s − 1) (s + 3) (s − 1) 2 s − 1 s + 3
. . . . .
.
Solution.

2
3s − 16s + 21
A = = 2.
s + 3 s=1
2
Move the term to the left hand side (LHS) and simplify:
(s − 1) 2

2
3s − 16s + 21 2 B C
− = + ⇒
2
(s − 1) (s + 3) (s − 1) 2 s − 1 s + 3
2
3s − 18s + 15 B C
= + .
2
. . . . (s − 1) (s + 3) s − 1 s + 3

33