Page 37 - 4811

Properties of Laplace Transform
.

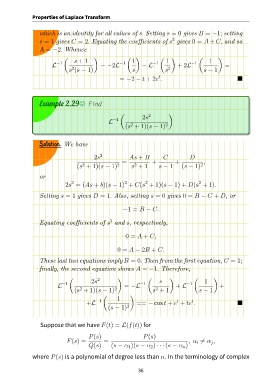
which is an identity for all values of s. Setting s = 0 gives B = −1; setting
2
s = 1 gives C = 2. Equating the coefficients of s gives 0 = A + C, and so
A = −2. Whence

( ) ( ) ( ) ( )
s + 1 1 1 1
−1 −1 −1 −1
L = −2L − L + 2L =
2
s (s − 1) s s 2 s − 1
t
= −2 − t + 2e .
. . . .
.

Example 2.29, Find

( 2 )
2s
−1
L
2
(s + 1)(s − 1) 2
.
. . . . .
Solution. We have

2s 2 As + B C D
= + + ,
2
2
(s + 1)(s − 1) 2 s + 1 s − 1 (s − 1) 2
or
2
2
2
2
2s = (As + b)(s − 1) + C(s + 1)(s − 1) + D(s + 1).
Setting s = 1 gives D = 1. Also, setting s = 0 gives 0 = B − C + D, or
−1 = B − C.

3
Equating coefficients of s and s, respectively,

0 = A + C,

0 = A − 2B + C.

These last two equations imply B = 0. Then from the first equation, C = 1;
finally, the second equation shows A = −1. Therefore,

( 2 ) ( ) ( )
2s s 1
−1 −1 −1
L = −L + L +
2
2
(s + 1)(s − 1) 2 s + 1 s − 1
( )
1
−1 t t
+L == − cos t + e + te .
(s − 1) 2
. . . .
Suppose that we have F(t) = L(f(t)) for
P(s) P(s)
F(s) = = , α ̸= α ,
j
i
Q(s) (s − α )(s − α ) · · · (s − α )
2
n
1
where P(s) is a polynomial of degree less than n. In the terminology of complex
36