Page 30 - 4811

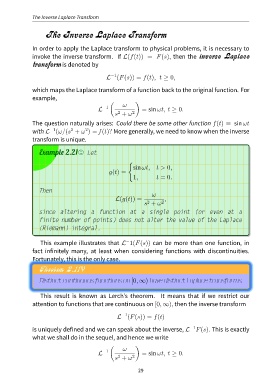
The Inverse Laplace Transform

The Inverse Laplace Transform

In order to apply the Laplace transform to physical problems, it is necessary to
invoke the inverse transform. If L(f(t)) = F(s), then the inverse Laplace

transform is denoted by

−1
L (F(s)) = f(t), t ≥ 0,

which maps the Laplace transform of a function back to the original function. For
example,
( )
ω
−1
L = sin ωt, t ≥ 0.
2
s + ω 2
The question naturally arises: Could there be some other function f(t) ≡ sin ωt
−1
2
2
with L (ω/(s + ω ) =f(t)? More generally, we need to know when the inverse
.
transform is unique.
Example 2.21, Let

{
sin ωt, t > 0,
g(t) =
1, t = 0.

Then
ω
L(g(t)) = ,
2
s + ω 2
since altering a function at a single point (or even at a
finite number of points) does not alter the value of the Laplace
(Riemann) integral.
. . . . .
This example illustrates that L 1(F(s)) can be more than one function, in
−
fact infinitely many, at least when considering functions with discontinuities.
Fortunately, this is the only case.
Theorem 2.11È

Distinct continuous functions on [0, ∞) have distinct Laplace transforms.
. . . . . . .
This result is known as Lerch’s theorem. It means that if we restrict our
attention to functions that are continuous on [0, ∞), then the inverse transform

−1
L (F(s)) = f(t)
−1
is uniquely defined and we can speak about the inverse, L F(s). This is exactly
what we shall do in the sequel, and hence we write

( )
ω
−1
L = sin ωt, t ≥ 0.
2
s + ω 2
29