Page 35 - 4811

Properties of Laplace Transform
.

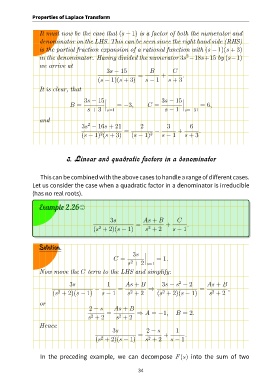
It must now be the case that (s − 1) is a factor of both the numerator and

denominator on the LHS. This can be seen since the right hand side (RHS)
is the partial fraction expansion of a rational function with (s − 1)(s + 3)
2
in the denominator. Having divided the numerator 3s −18s+15 by (s−1)
we arrive at
3s − 15 B C
= + .
(s − 1)(s + 3) s − 1 s + 3

It is clear, that

3s − 15 3s − 15
B = = −3, C = = 6,
s + 3 s=1 s − 1 s=−31

and
2
3s − 16s + 21 2 3 6
= − + .
2
(s − 1) (s + 3) (s − 1) 2 s − 1 s + 3
. . . .

3. Linear and quadratic factors in a denominator

This can be combined with the above cases to handle a range of different cases.
Let us consider the case when a quadratic factor in a denominator is irreducible
.
(has no real roots).

Example 2.26,

3s As + B C
= + .
2
2
(s + 2)(s − 1) s + 2 s − 1
. . . . .
.
Solution.

3s
C = = 1.
2
s + 2 s=1
Now move the C term to the LHS and simplify:

2
3s 1 As + B 3s − s − 2 As + B
− = ⇒ = ,
2
2
2
2
(s + 2)(s − 1) s − 1 s + 2 (s + 2)(s − 1) s + 2
or
2 − s As + B
= ⇒ A = −1, B = 2.
2
2
s + 2 s + 2
Hence
3s 2 − s 1
= + .
2
2
(s + 2)(s − 1) s + 2 s − 1
. . . .
In the preceding example, we can decompose F(s) into the sum of two

34