Page 22 - 4811

Derivatives

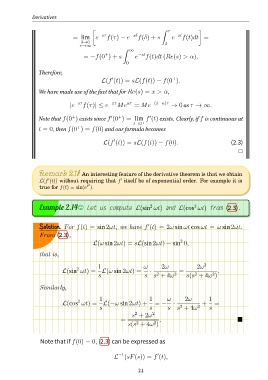
[ ∫ τ ]
= lim e −sτ f(τ) − e −sδ f(δ) + s e −st f(t)dt =
δ→0 δ
τ→∞
∫
∞
+
= −f(0 ) + s e −st f(t)dt (Re(s) > α).
0
Therefore,
+
′
L(f (t)) = sL(f(t)) − f(0 ).
We have made use of the fact that for Re(s) = x > α,

|e −sτ f(τ)| ≤ e −xτ Me ατ = Me −(x−α)τ → 0 as τ → ∞.

+
+
′
Note that f(0 ) exists since f (0 ) = lim f (t) exists. Clearly, if f is continuous at
′
t→0 +
+
t = 0, then f(0 ) = f(0) and our formula becomes
′
L(f (t)) = sL(f(t)) − f(0). (2.3)
2
. . . .

Remark 2.1E An interesting feature of the derivative theorem is that we obtain
L(f (t)) without requiring that f itself be of exponential order. For example it is
′
′
2
t
true for f(t) = sin(e ).
.
. . . . .
2
2
Example 2.14, Let us compute L(sin ωt) and L(cos ωt) from (2.3).
. . . . .
.
Solution. For f(t) = sin 2ωt, we have f (t) = 2ω sin ωt cos ωt = ω sin 2ωt.
′
From (2.3),
2
L(ω sin 2ωt) = sL(sin 2ωt) − sin 0,

that is,

1 ω 2ω 2ω 2
2
L(sin ωt) = L(ω sin 2ωt) = · = .
2
2
2
s s s + 4ω 2 s(s + 4ω )
Similarly,
1 1 ω 2ω 1
2
L(cos ωt) = L(−ω sin 2ωt) + = − · + =
2
s s s s + 4ω 2 s
2
s + 2ω 2
= .
2
2
s(s + 4ω )
. . . .
Note that if f(0) = 0, (2.3) can be expressed as

−1
′
L (sF(s)) = f (t),
21