Page 27 - 4811

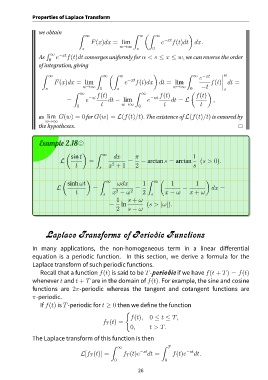
Properties of Laplace Transform

we obtain
∫ ∫ (∫ )
∞ w ∞
F(x)dx = lim e −xt f(t)dt dx.
s w→∞ s 0
∫ ∞
As e −xt f(t)dt converges uniformly for α < s ≤ x ≤ w, we can reverse the order
0
of integration, giving

∫ ∫ (∫ ) ∫ w
∞ ∞ w ∞ e −xt
F(x)dx = lim e −xt f(t)dx dt = lim f(t) dt =

s w→∞ 0 s w→∞ 0 −t s
∫ ∫ ( )
∞ f(t) ∞ f(t) f(t)
= e −st dt − lim e −wt dt = L ,
0 t w→∞ 0 t t

as lim G(w) = 0 for G(w) = L(f(t)/t). The existence of L(f(t)/t) is ensured by
w→∞
. . . . the hypotheses. 2
.

Example 2.18,
( ) ∫ ∞
sin t dx π 1
L = = − arctan s = arctan (s > 0).
2
t s x + 1 2 s

( ) ∫ ∞ ∫ ∞ ( )
sinh ωt ωdx 1 1 1
L = = − dx =
2
t s x − ω 2 2 s x − ω x + ω
1 s + ω
= ln (s > |ω|).
2 s − ω
. . . . .

Laplace Transforms of Periodic Functions

In many applications, the non-homogeneous term in a linear differential
equation is a periodic function. In this section, we derive a formula for the
Laplace transform of such periodic functions.

Recall that a function f(t) is said to be T-periodic if we have f(t + T) = f(t)
whenever t and t + T are in the domain of f(t). For example, the sine and cosine
functions are 2π-periodic whereas the tangent and cotangent functions are

π-periodic.
If f(t) is T-periodic for t ≥ 0 then we define the function
{
f(t), 0 ≤ t ≤ T,
f (t) =
T
0, t > T.

The Laplace transform of this function is then

∫ ∫
∞ T
L[f (t)] = f (t)e −st dt = f(t)e −st dt.
T
T
0 0
26