Page 21 - 4811

Properties of Laplace Transform

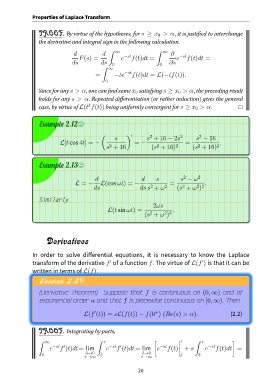
PROOF. By virtue of the hypotheses, for s ≥ x > α, it is justified to interchange
0
the derivative and integral sign in the following calculation.

∫ ∞ ∫ ∞
d d ∂
F(s) = e −st f(t)dt = e −st f(t)dt =
ds ds 0 0 ∂s
∫
∞
= −te −st f(t)dt = L(−tf(t)).
0
Since for any s > α, one can find some x satisfying s ≥ x > α, the preceding result
0
0
holds for any s > α. Repeated differentiation (or rather induction) gives the general
k
. . . . case, by virtue of L(t f(t)) being uniformly convergent for s ≥ x > α. 2
.
0
Example 2.12,

( ) ′ 2 2 2
s s + 16 − 2s s − 16
L[t cos 4t] = − s + 16 = − (s + 16) 2 = (s + 16) 2 .
2
2
2
.
. . . . .
Example 2.13,
2
d d s s − ω 2
L = − L(cos ωt) = − = .
2
2 2
2
ds ds s + ω 2 (s + ω )
Similarly,
2ωs
L(t sin ωt) = .
2
2 2
(s + ω )
. . . . .

Derivatives

In order to solve differential equations, it is necessary to know the Laplace
transform of the derivative f of a function f. The virtue of L(f ) is that it can be
′
′
written in terms of L(f).
Theorem 2.5È

(Derivative Theorem). Suppose that f is continuous on (0, ∞) and of
exponential order α and that f is piecewise continuous on [0, ∞). Then

+
L(f (t)) = sL(f(t)) − f(0 ) (Re(s) > α). (2.2)
′
. . . . . . .
PROOF. Integrating by parts,

∫ ∫ τ [ τ ∫ τ ]
∞

e −st ′ e −st ′ −st f(t) + s e −st f(t)dt =
f (t)dt= lim e
f (t)dt= lim

δ→0
δ→0
. . . . 0 τ→∞ δ τ→∞ δ δ
20