Page 26 - 4811

Integration
.

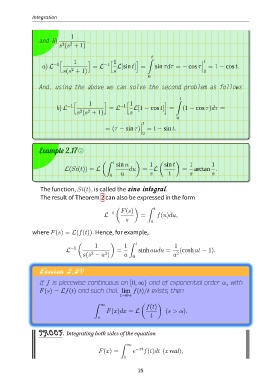
1
and b) .
2
2
s (s + 1)
t
∫
[ 1 ] [ 1 ] t
−1 −1
a) L = L L[sin t] = sin τdτ = − cos τ = 1 − cos t.
2
s(s + 1) s 0
0
And, using the above we can solve the second problem as follows

t
∫
[ 1 ] [ 1 ]
−1 −1
b) L = L L[1 − cos t] = (1 − cos τ)dτ =
2
2
s (s + 1) s
0
t

= (τ − sin τ) = t − sin t.
.
. . . . . 0
Example 2.17,
t
(∫ ) ( )
sin u 1 sin t 1 1
L(Si(t)) = L du = L = arctan .
u s t s s
0
. . . . .
The function, Si(t), is called the sine integral.
The result of Theorem 2 can also be expressed in the form

( ) ∫ t
F(s)
−1
L = f(u)du,
s 0

where F(s) = L(f(t)). Hence, for example,

( ) ∫ t
1 1 1
−1
L = sinh audu = (cosh at − 1).
2
2
s(s − a ) a a 2
0
Theorem 2.9È

If f is piecewise continuous on [0, ∞) and of exponential order α, with
F(s) = Lf(t) and such that lim f(t)/t exists, then
t→0+

∫ ( )
∞
f(t)
F(x)dx = L (s > α).
s t
. . . . . . .

PROOF. Integrating both sides of the equation

∫
∞
F(x) = e −xt f(t)dt (x real),
. . . . 0

25