Page 39 - 4560

where  ,  ,  - angles of resistant cross-section rotation
, n n 1 nn , n n 1
of one-span beam of equivalent systeme on n й resistance to
the action of the points M n 1 1 , M  1, M n 1 1 , but  -
n
пр
from the effects of external loading.
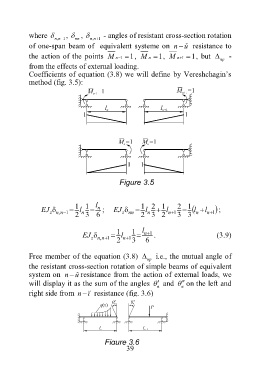
Coefficients of equation (3.8) we will define by Vereshchagin’s
method (fig. 3.5):

Figure 3.5

1 1 l 1 2 1 2 1
EJ   l  n ; EJ   l  l   ll ;
n
1
,n
z
2 n 3 6 z nn 2 n 3 2 n 1  3 3 n n 1 

1 1 l n  1
EJ z  n ,n 1   l n  1 3  6 . (3.9)
2

Free member of the equation (3.8)  i.e., the mutual angle of
пр
the resistant cross-section rotation of simple beams of equivalent
system on n й resistance from the action of external loads, we
will display it as the sum of the angles  and  on the left and
n n
right side from n ї resistance (fig. 3.6)

Figure 3.6
39