Page 38 - 4560

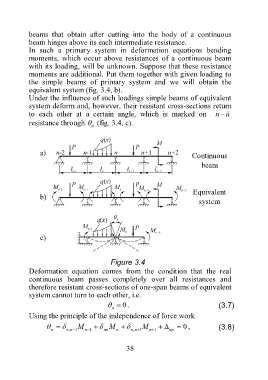
beams that obtain after cutting into the body of a continuous
beam hinges above its each intermediate resistance.
In such a primary system in deformation equations bending
moments, which occur above resistances of a continuous beam
with its loading, will be unknown. Suppose that these resistance
moments are additional. Put them together with given loading to
the simple beams of primary system and we will obtain the
equivalent system (fig. 3.4, b).
Under the influence of such loadings simple beams of equivalent
system deform and, however, their resistant cross-sections return
to each other at a certain angle, which is marked on n й
resistance through  (fig. 3.4, c).
n

a) Continuous
) beam

Equivalent
b)
system

c)

Figure 3.4
Deformation equation comes from the condition that the real
continuous beam passes completely over all resistances and
therefore resistant cross-sections of one-span beams of equivalent
system cannot turn to each other, i.e.
  0. (3.7)
n
Using the principle of the independence of force work
   M   M   M    0 , (3.8)
n , n n 1 n 1 nn n , n n 1 n 1 np

38