Page 35 - 4560

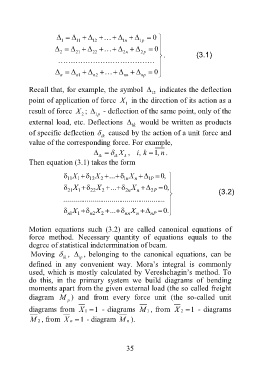
           0 
1 11 12 1n 1p

           0 
2 21 22 2n 2 p  . (3.1)


           0
n 1 n n 2 nn np 

Recall that, for example, the symbol  indicates the deflection
12
point of application of force X in the direction of its action as a
1
result of force X ;  - deflection of the same point, only of the
2 1p
external load, etc. Deflections  would be written as products
ik
of specific deflection  caused by the action of a unit force and
ik
value of the corresponding force. For example,
   X , ,i k  1, n .
ik ik k
Then equation (3.1) takes the form

 X 1   X 2 ...   X n   1P  ,0 
12
11
1n

 X 1   X 2 ...   X n   2P  ,0 
21
2n
22
 (3.2)
.......... .......... .......... .......... .......... ...

 X   X ...   X    .0 
1 n 1 2 n 2 nn n nP 

Motion equations such (3.2) are called canonical equations of
force method. Necessary quantity of equations equals to the
degree of statistical indetermination of beam.
Moving  ,  , belonging to the canonical equations, can be
ik ip
defined in any convenient way. Mora’s integral is commonly
used, which is mostly calculated by Vereshchagin’s method. To
do this, in the primary system we build diagrams of bending
moments apart from the given external load (the so called freight
diagram M ) and from every force unit (the so-called unit
p
diagrams from X 1 1 - diagrams M , from X 2 1 - diagrams
1
M 2 , from X  1 - diagram M ).
n
n

35