Page 37 - 4560

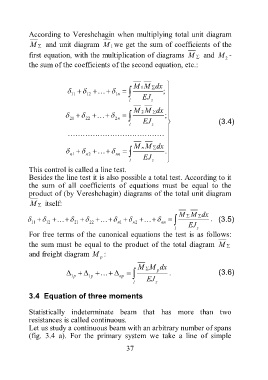
According to Vereshchagin when multiplying total unit diagram
M  and unit diagram M we get the sum of coefficients of the
1
first equation, with the multiplication of diagrams M  and M -
2
the sum of the coefficients of the second equation, etc.:

M 1M dx 

       ; 
1n 
11 12 EJ
l z 

M 2M dx

      2n  ;


21 22 EJ (3.4)
l z 



M n M dx 


      nn  .
1 n n 2 EJ
l z  
This control is called a line test.
Besides the line test it is also possible a total test. According to it
the sum of all coefficients of equations must be equal to the
product of (by Vereshchagin) diagrams of the total unit diagram
M  itself:
M M dx


                nn  . (3.5)

11 12 21 22 1 n n 2 EJ
l z
For free terms of the canonical equations the test is as follows:
the sum must be equal to the product of the total diagram M 
and freight diagram M :
p
M M dx

       p . (3.6)
1p 1p np  EJ
l z

3.4 Equation of three moments

Statistically indeterminate beam that has more than two
resistances is called continuous.
Let us study a continuous beam with an arbitrary number of spans
(fig. 3.4 a). For the primary system we take a line of simple
37