Page 30 - 4560

1 
    x dx ,
f x f 2
l
(2.14) where one of the functions, for
f
example   x , expresses the internal
1
power factor of the load unit and the
second function f   x - from a given
2
load.
 
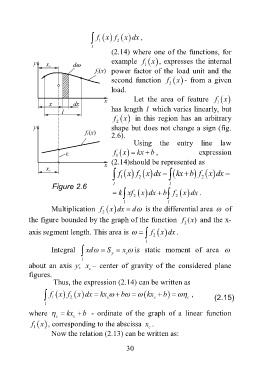
Let the area of feature f x
1
has length l which varies linearly, but
f   x in this region has an arbitrary
2
shape but does not change a sign (fig.
2.6).
Using the entry line law
f   x  kx b , expression
1
(2.14)should be represented as
f x f 2  kx b f x dx    
    x dx 
1 
2
Figure 2.6 l l

 k xf 2   x dx b f 2    x dx .
l l
f
Multiplication   x dx  d is the differential area  of
2
the figure bounded by the graph of the function f ( )x and the x-
2
axis segment length. This area is   f 2    x dx .
l
Integral  xd  S  x  is static moment of area 
y
c
l
about an axis у; x – center of gravity of the considered plane
c
figures.
Thus, the expression (2.14) can be written as
    x dx 
f x f 2 kx   b  kx   b   , (2.15)
1 
c
c
c
l
b
where   kx  - ordinate of the graph of a linear function
c c
f   x , corresponding to the abscissa x .
1 c
Now the relation (2.13) can be written as:
30