Page 68 - 4234

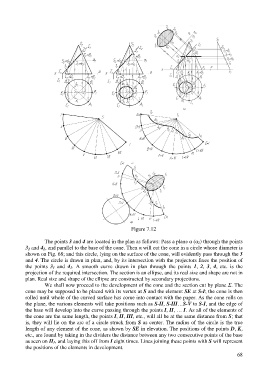
Figure 7.12

The points 3 and 4 are located in the plan as follows: Pass a plane α (α 2) through the points
3 2 and 4 2, and parallel to the base of the cone. Then α will cut the cone in a circle whose diameter is
shown on Fig. 68; and this circle, lying on the surface of the cone, will evidently pass through the 3
and 4. The circle is drawn in plan, and, by its intersection with the projectors fixes the position of
the points 3 1 and 4 1. A smooth curve drawn in plan through the points 1, 2, 3, 4, etc. is the
projection of the required intersection. The section is an ellipse, and its real size and shape are not in
plan. Real size and shape of the ellipse are constructed by secondary projections.
We shall now proceed to the development of the cone and the section cut by plane Σ. The
cone may be supposed to be placed with its vertex at S and the element SE at S-I; the cone is then
rolled until whole of the curved surface has come into contact with the paper. As the cone rolls on
the plane, the various elements will take positions such as S-II, S-III…S-V to S-I, and the edge of
the base will develop into the curve passing through the points I, II, … I. As all of the elements of
the cone are the same length, the points I, II, III, etc., will all be at the same distance from S; that
is, they will lie on the arc of a circle struck from S as center. The radius of the circle is the true
length of any element of the cone, as shown by SE in elevation. The positions of the points D, K,
etc., are found by taking in the dividers the distance between any two consecutive points of the base
as seen on Π 1, and laying this off from I eight times. Lines joining these points with S will represent
the positions of the elements in development.
68